Set Relations

Sunday, 10 March 2019

8:37 PM

A relation  from a set  to a set  is a subset of

If  then "a is related to b (by R)" ->

 

 

 

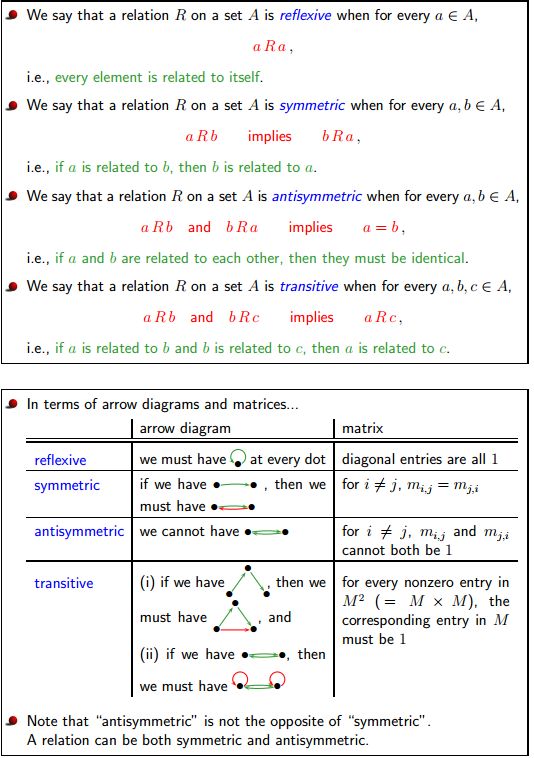
A relation R on a set A is reflexive when

(every element is related to itself)

 

A relation R on a set A is symmetric if  and

(if a is related to b, b is related to a)

 

A relation R on a set A is antisymmetric if  and  results in

(if a and b are related, they must be identical)

(// no points are symmetric //)

 

A relation R on a set A is transitive if  and  implies

(if a is related to b, and b is related to c, then a is related to c)

 

Machine generated alternative text: S We say that a relation R on a Set A is reflexive when for every a A, aRa, , every element is related to itself. S We say that a relation R on a set A is symmetric when for every a, b € A, a R b implies b R a , i.e., if a is related to b, then b is related to a. S We say that a relation R on a set A is antisymmetric when for every a, b € A, a b and b Ra implies i.e., if a and b are related to each other, then they must be identical. S We say that a relation R on a set A is transitive when for every a, b, c € A, a R b and b Rc implies if a is related to b and b is related to c, then a is related to c. S In terms of arrow diagrams and matrices.. arrow.' diagram reflexive symmetric antisymmetric transitive we must have at every dot if we have , then we must have we cannot have (i) if we have , then we must have , and matrix diagonal entries are all 1 for i # j, m, j = mj,i for i J, m, j and mj cannot both be 1 for every nonzero entry in AP ( Al x M), the corresponding entry in M must be 1 (ii) if we have then we must have S Note that "antisymmetric" is not the opposite of "symmetric". A relation can be both symmetric and antisymmetric.

 

Created with Microsoft OneNote 2016.