Sinusoid-phasor Transformation

Monday, 17 September 2018

1:45 PM

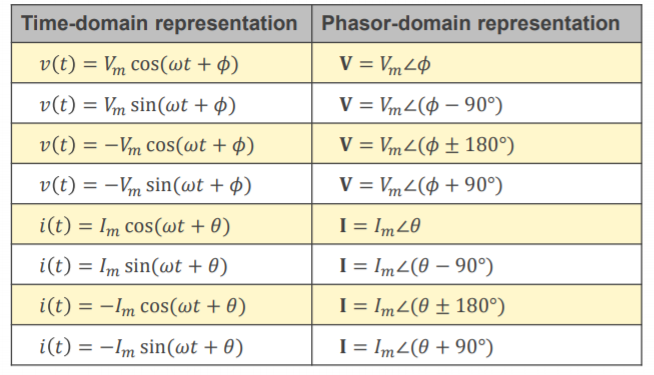
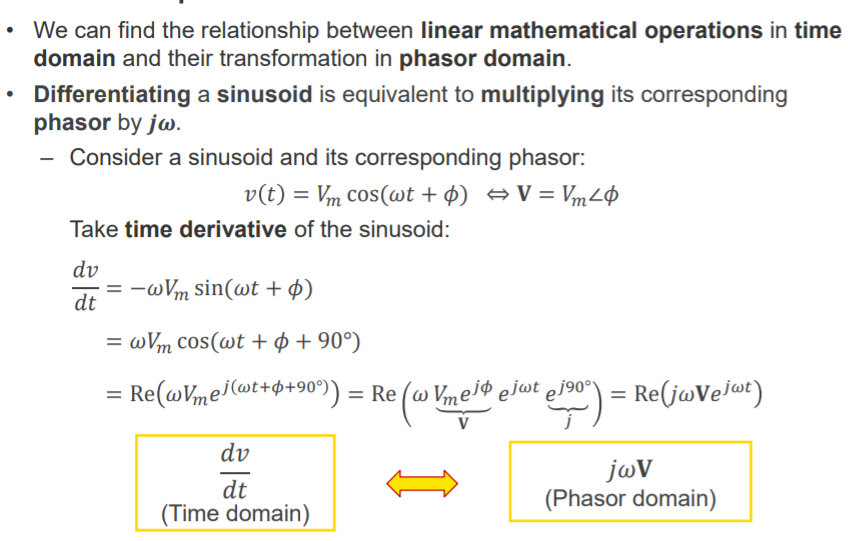
Phasors are defined in cosine form, so other sinusoid forms need to be converted to their cosine form

 

Machine generated alternative text: Time-domain representation v(t) = Vm cos(wt + (P) v(t) = Vm sin(wt + (P) v(t) = —Vm cos(wt + 4) v(t) = —Vim sin(wt + 4) i(t) = 1m cos(wt + O) i(t) = 1m sin(wt + 9) i(t) = —1m cos(wt + 9) i(t) — — —1m sin(wt + O) Phasor-domain representation V = VmL(Ø — 900) V = VmL(Ø ± 1800) V = vmL@ + 900) 1 = 1mL(9 — 900) 1 = 1mL(9 ± 1800) 1 = 1mL(9 + 900)

 

Machine generated alternative text: • We can find the relationship between linear mathematical operations in time domain and their transformation in phasor domain. Differentiating a sinusoid is equivalent to multiplying its corresponding phasor by jü). Consider a sinusoid and its corresponding phasor: v(t) = Vm cos(ot+ 4) V = VmLØ Take time derivative of the sinusoid: dv — —wVm sin(ot + 4) dt ¯ = (DVm cos(wt + + 900) = = Re Vmejqb ejwt ej900 = Re(jwVejwt) v dv dt (Time domain) jov (Phasor domain)

 

 

Created with Microsoft OneNote 2016.